Physics defines speed as "distance traveled divided by the time of travel". When looking at "speed", zero means that an object is at rest (the distance traveled is zero). The interval size is chosen based on the units of distance and time. In the metric system, the speed of a vehicle is measured in kilometres per hour (km/h). In the Imperial system speed of a vehicle is measured in miles per hour (mph).
A comparison of the two ratio scales is shown in the illustration below.
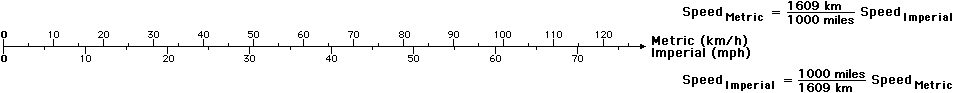
Equal differences in any two speeds on one scale will result in equal differences on the other scale.
- 20 km/h is equivalent to 12.43 mph
- 35 km/h is equivalent to 21.75 mph
- 55 km/h is equivalent to 34.18 mph
- 70 km/h is equivalent to 43.50 mph

Note that 35 km/h - 20 km/h = 70 km/h - 55 km/h (both are a difference of 15 km/h).
Note that 21.75 mph - 12.43 mph = 43.50 mph - 34.18 mph (both are a difference of 9.52 mph).
A difference of 15 km/h is equivalent to a difference of 9.52 mph, regardless of where you are on either scale.

The ratio of any two speeds on one scale will be equivalent to the ratio of two equivalent speeds on another scale.
- 42 km/h is equivalent to 26.103 mph
- 84 km/h is equivalent to 52.206 mph

The ratio of 84 km/h to 42 km/h is 2.
The ratio of 52.206 mph to 26.103 mph is also 2.
Twice as fast on either scale has the same meaning since the location of zero represents the same thing on both scales.
If it is desired to know how how fast it is if it is twice as fast as 42 km/h, multiply 42 km/h by 2.
If it is desired to know how how fast it is if it is twice as fast as 26.103 mph, multiply 26.103 mph by 2.
Canadian cars usually display speed in both Metric and Imperial units, as illustrated in the sample display below.
